These Dice Are Driving Me Crazy! – A Guest Blog
As stated in the Dice Hate Me charter, bad dice karma isn’t just a clever name, it’s a way of life around these parts. It seems that wherever Dice Hate Me appears at conventions or gaming events, there are gamers who immediately identify with the sentiment set forth by the brand, and then there are a select few who laugh, but brush aside the mention of such foolishness. Well, I hate to say it to those latter folk, but – I told you so. Case in point, the following brilliant post about dice-rolling statistics by FrontRangeGamer from Front Range Gamers. Lest you think that this guy is just blowing about some hot air, he dabbles in quantum matters at the National Institute of Standards and Technology which, for us laymen, means he knows a thing or two about probability. As for me, the following involves a lot of math. For those of you who know me well, you know that I’m not exactly a math guy. In the words of Han Solo: “Never tell me the odds.” In this case, I’ll make an exception – this brilliant breakdown of how the dice may, indeed, hate me was too good not to share with all of you. So sit back, get your math on, and take a look at dice karma from a scientific perspective.
 I played a game of Settlers of Catan recently, and was frustrated by the dice rolling. I’m sure everybody has had this experience, when it seems like statistics have gone out the window. You start to think that you should have built on that spot with an 11 instead of the spot with the 6, because that what keeps coming up. It turns out that this is exactly what statistics says should happen, people just aren’t very good at thinking about randomness.
I played a game of Settlers of Catan recently, and was frustrated by the dice rolling. I’m sure everybody has had this experience, when it seems like statistics have gone out the window. You start to think that you should have built on that spot with an 11 instead of the spot with the 6, because that what keeps coming up. It turns out that this is exactly what statistics says should happen, people just aren’t very good at thinking about randomness.
I’ll start with a quick explanation of how Settlers works. Each turn you roll two dice with six sides (2d6), then everybody gets resources if their settlements or cities border land that has that number on it. There are number tiles from 2-12, with little dots on them that tell you how often that number should happen. So 2 has a single dot, and 6 has five dots. A good settlement might a 6, a 5 and a 4 for a total of 12 dots.
We’re going to take a look at what statistics says should happen in games like this. First lets just look at rolling 2d6. The fundamental idea behind trying to figure out what should happen, is that every time you roll a dice, each number on that dice is equally likely. It will help to understand if the dice are different colors, orange and black. Every time I roll, the orange die will randomly pick a number from 1-6 and the black dice will independently pick a random number from 1-6. Every number is equally likely for any given die, but if you are interested in the total of both die, then some numbers are more likely than others.
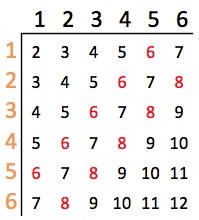
All the possible combinations from rolling two six sided dice. The black die will randomly select a column, and the orange die will randomly select a row, so you can see that each entry in the table is equally likely.
The table shows the results from two dice, black and orange, and their sums. Imagine rolling the black die to pick a column, then rolling the orange die to pick a number from that column, and you will see that each entry in the table is equally likely. So the probability of getting a certain value in a 2d6 roll is the number of times that value show up in the table, divided by how many numbers are in the table (36). We can see that 2 should happen 1 time in 36 rolls, and 7 should happen 6 in 36 rolls. The dots on the number tiles in Settlers of Catan are exactly how many time that number shows up in this table. So 2 has only one dot, while 6 and 8 have 5 dots. So if you add up all the dots next to your settlement, you should get one resource per 36/(number of dots) turns.
Probabilities like this don’t tell you exactly what is going to happen, they can only tell you about how likely it is that things will happen. If you roll these dice 6000 times, you will get seven about 1000 times. But you might get 1032 or 973 or 1001, you can’t know in advance. The typical variation in how many sevens you roll will be roughly the square root of how many you expected to roll based on the probability. The square root of 1000 is about 30, so you will typically get 1000 sevens to within 30 or so. If you only roll these dice 36 times, you expect to get 1 two. The square root of 1 is 1. This means you expect to have +/- 100% or more variations in how many twos you actually get. The point is, if you look at a lot of dice rolls, the variations will be a small percent of the total rolls. But if you look at just a few dice rolls, the variations will be almost as big as the total rolls. And in board games we almost always look at just a few dice rolls.
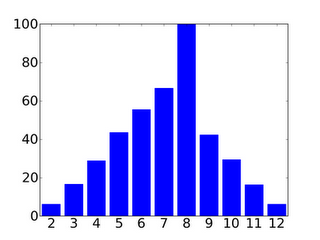
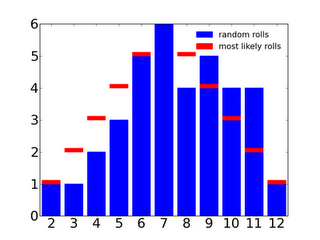
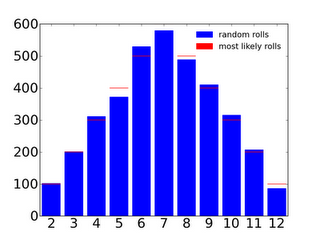
I ran some simulations to give you an idea of what this means. The next two pictures are simulations of rolling 2d6 36 times. This is like going through 9 turns in a 4 player game of Settlers. The red lines show how many times each number is expected to come up, and the blue bars show how many times it actually did come up. You can see that there is huge variation in each set of 9 turns. In case you haven’t seen this type of plot, the x-axis shows the value of the dice rolls, and the y-axis shows how many times it happened.
If you look at a lot more rolls at a time, the results look much more like the expected outcome. Here is a simulation of 3600 rolls, which is something like 10-20 full games of Settlers. The variations are a much smaller fraction of the total rolls.
The worst is when you have the resource you need on a good number like an 8, and your opponent crushes you because 11 just keeps coming up. In small sets of rolls, it is actually pretty common for a crappy number to come up more often than a better number. Here I ran some simulations to find out how often numbers come up more often than 5. If you look at sets of 36 rolls, 11 comes up more than 8 over 20% of the time!
What % of the time a number comes up at least as often as 8 in sets of 36 rolls. If you look at a small number of dice rolls, any number can come up more often than 8, even 2 or 12. Its not even very rare.
If instead you look at 3600 rolls, you can basically be guaranteed that the better numbers will come up more often.
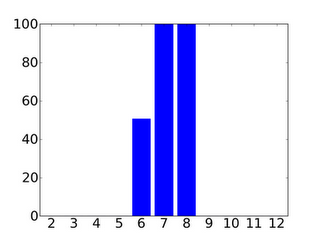
What % of the time a number comes up at least as often as 8 in sets of 3600 rolls. Basically, 7 always come up more often than 8, 6 comes up more often about half the time, and nothing else ever comes up more often. Just like we expect, but it is only like this if you look at lots of rolls at a time.
So now we can see why the dice drive us crazy so often. If you look at small sets of rolls, statistics say that weird stuff will happen. Uncommon rolls will come up more often than common rolls, regularly. It would actually be weird if that didn’t happen! When did the dice screw you over? Or make the game for you? Leave a comment.
P.S. – If you want to know more about the statistics of random events, look up the Poisson Distribution. Can you figure out where I fudged on the math to make the story simpler?
Related posts:
- Guest Impressions: GenCon 2011
- Dice Hate Me Game of the Year Awards 2010
- Dice Hate Me at the Bookstore: The Games Bible
- The 2010 Dice Hate Me Holiday Gift Guide
- Enter the Rabbit – A Cookie Fu Review



Nice. I haven’t had a solid math-y article in a while.
Great article that explains all that complex math, plus the graphs a bonus.
Nice article and a good point about how fewer rolls produce more variation. When tinkering with game design I often use this site:
http://www.pvv.ntnu.no/~bcd/SR/dicerollcalc.html
It was designed for the Shadowrun RPG and does the math for you.
Steve”Tell me the odds- I’ll beat them anyway…”Avery
It’s fun to see my article on a bigger site.
I’m thinking of doing an article on dice roll superstitions. The one that comes up jokingly in my group is “pre roll the ones”. I’m wondering if anybody has more serious dice rolling superstitions?
Oh, I have quite a few dice roll superstitions. I like to “charge” my dice if I need a big roll – I leave them sitting with the six (or highest) side face up. That puts all the weight on the bottom on the ones so the six comes up more often. 😉 I also like to have my own set of dice during a game when I can, to offset any bad dice karma (well, worse than my own, which is rare).
Thanks for the article. I could use to see more Statistics articles on board games.
Best,
Phil
You have been able to articulate what I’ve known intuitively as a game player and theoretically as a mathematician – that there is a difference between the expected value of dice outcomes and the reality of a small number of trials. But the obviously tricky part about a game like Settlers of Catan is that, even if I know that I won’t get the “normal” distribution in the game, I still don’t know which outliers will outperform the sixes and the eights. So I still have to play the odds.
Some people like to mitigate the odds by placing settlements on a wide variety of numbers – for example, not starting both settlements on sixes. My belief is that there’s no difference from a probability outcome standpoint, but since there’s a game consequence to having too many cards in your hand (because the thief can take half of them), it does make sense to avoid windfalls if you can spread your opportunities across a larger variety of outcomes.
As for dice superstitions, in my group, we are definitely superstitious about which dice to roll when – usually having to do with the color of the dice and how they’ve been performing in that game. I posted recently about a game of Firestorm Armada that involved a lot of dice-rolling, and we definitely found ourselves avoiding some colors and preferring others. Some of my best battleship attacks came when I adhered rigorously to my color preferences. When we play WarZone, one player in our group has an inordinate fondness for “the blood die,” a large green 20-sided die with red numbers. He thinks it’s a killer; the rest of us think it’s bad luck and the source of numerous failed attacks.
For an otherwise very analytic group, it is funny to see how superstitious we are about dice.